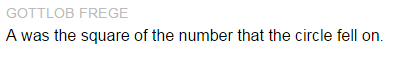I recently submitted a proposal to speak at NCTM on using Desmos to create opportunities for students to be right and wrong in different, interesting ways. As I have used Desmos, this is the design principle that continues to stick out the most for me. After seeing My Favorite No a few years back I have tried to use incorrect student responses to drive my instruction as much as possible. Students see the teacher more interested in their thinking if they are wrong. It validates all student thinking and encourages students to put their ideas out there. Desmos activity builder is a tool that helps ask these types of questions. It also easily collects, organizes, and displays student responses to help encourage whole-class discussions.
Taking the advice of Dan Meyer and Robert Kaplinsky, I proposed something that would inspire me to research it. That research began today as I ran a Desmos activity that I created to introduce students to the equations of circles. What follows are a few reflections on how things went.
Using Student Responses to Drive Instruction
In this activity students were to adjust sliders for different parts of the equation of a circle and observe what happens to the graph. They would then apply what they observed to match different circles. I also added a few error analysis questions and marble slides at the end for practice.
The first opportunity for students to be right and wrong in different, interesting ways is slide 2 where students were given the opportunity to see what part of the equation connects to the radius of the circle. I used the variable a to represent "r^2" in the equation. I did not want students to automatically think about the radius, and I also wanted them to notice the equation did not contain the direct value of the radius - it contains the radius squared. I also left out the negatives for h and k because I wanted students to see the "opposite" movements. After going over the activity, we derived the formula using the Pythagorean Theorem so that students could see why the center coordinates have the opposite sign of the values in the equation.
Below is the order in which I shared responses for slide 2 and a few notes on the discussion we had. By giving this assignment at the end of class, I was able to take my time reading the responses and organizing the progression I wanted to use in sharing them with the class. Now that I have run this activity, I know what to look for so I would be more comfortable doing that on the fly during class.
Several students observed a change in the size and went no further. These responses speak to the accessibility of the question for all levels. You don't need to know any math to make an observation. It was not necessary to use any formal notation or vocabulary. Students could just share what they saw.
The majority of students went further by identifying one of the above measurements as increasing or decreasing as the value of a increased or decreased.
There were multiple responses like the one above that mentioned the midpoint of the circle. In my mind, this is a really interesting response. As someone teaching geometry for the first time this year, I would not have planned to mention midpoint in a lesson on circles. It makes perfect sense to see the center as a midpoint of the diameter of a circle though. These students are using what they already know. Giving students the chance to play and make observations allowed me to center our class discussion on their prior knowledge and own language to develop new vocabulary.
A few students went even further and made the connection that the specific value of a was the same as the radius squared. I found the first two responses above interesting because they did not directly say radius. Once again, our discussion could start with those descriptions and work towards understanding exactly what they meant. Allowing students to start the discussion created a need for a common language and vocabulary.
Activity Design - Student Thinking
As I read through all the responses, I came across the following incorrect response on slide 16:
Once I saw this response, I went back and looked at slide 13 to find this response:
This student admits to "blindly" moving the a slider until he got the size of the circles to match. He did not stop and notice the direct connection between the value of a and the measure of the radius. These responses demonstrate the importance of adding slides to activities that force students to stop and explain their thinking. Having students identify errors also encourages them to revisit previous slides to try and make connections that they might not have noticed the first time through he activity.
Activity Design - Debriefing
The first version of this activity had slides 16 and 17 as short answer slides. I quickly realized that making it a multiple choice question with the option to have students explain would serve me better when debriefing the activity. As a multiple choice question, the responses on the teacher dashboard would be organized based on the choice the student selected. I am thankful for the design by Desmos that does not force me to choose multiple choice OR short answer. I can easily have students select an answer from the given choices AND explain their thinking.